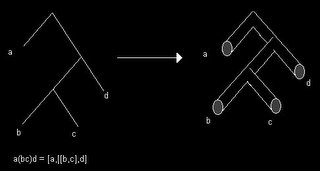
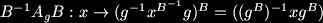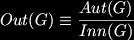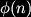First, there have been a number of introductory posts on the Barrett-Connes standard model spectral triple over at the n-category cafe, in particular see posts
I,
II,
III and
IV. Second John Barrett has been talking about his approach to finding the appropriate spectral triple in Cambridge yesterday, his paper, "
A Lorentzian version of the non-commutative geometry of the standard model of particle physics" appeared on the arxiv on the same day as Connes' and suggested identical alterations, and while I have been feverishly attacking my thesis, Alejandro Rivero attended the talk and has made some comments about it on
physics forums. He also has uploaded his
notes from the talk, but these are a little hard to read. Also the
Newton Institute have audio of all the talks from last week's workshop for you to enjoy
here.
On a different note, a while ago I attended the
Winter School on the Attractor Mechanism in Frascati but was unable to write up much about it due to being very busy. Well
Per Kraus, who gave a set of talks at the school has helped me out by publishing his lecture notes on the archive as
"Lectures on black holes and the AdS3/ CFT2 correspondence". Thank-you Per!
You have probably heard of
Penrose tilings,
Penrose diagrams,
Penrose limits,
Penrose triangles and even a
Penrose staircase, well last week, during
Roger Penrose's talk at the
Noncommutative Geometry Workshop, I heard a little about a Penrose Universe. (In my picture it looks like Roger Penrose is keeping the audience entertained with his shadow puppet routine)

It is a peculiar thing and represents Penrose's approach to understanding the second law of thermodynamics, that entropy increases, on a cosmological scale. Penrose points out that there is a contradiction in the entropy increase picture of the big bang, which is that the background radiation matches a model that is in thermal equilibrium. Such a state is just about the highest entropy state you can imagine for a system. Almost any other distribution besides uniform would result in a smaller entropy. The Big Bang picture requires the restriction of phase space at early time and the consequence that entropy ought to be tiny at the Big Bang. Penrose wonders how to resolve this contradiction, and seeks to resolve it by separating the entropy of the universe into that arising from matter (the eneergy momentum tensor) and that encoded in gravitational degrees of freedom (the Weyl curvature, see below).
In a nutshell it is a universe without a big crunch, and if taken to the extreme limit without a big bang, but which gives the features of energy density fluctuations in the background radiation. Penrose suggested his
Weyl curvature hypothesis in 1979 as a physical origin of the increasing entropy of the universe with time. The
Weyl curvature tensor is the traceless part of the Riemann curvature, i.e. the parts which when contracted upon two indices give zero for the Ricci (two-form) tensor. To quote Penrose's description,
"In Einstein’s theory the Ricci curvature R_{ab} is directly determined by the gravitational sources, via the energy-momentum tensor of matter (analogue of the charge-current vector J_{a} in Maxwell’s electromagnetic theory) and the remaining part of the space-time Riemann curvature, namely the Weyl curvature C_{abcd}, describes gravitational degrees of freedom (analogue of the field tensor F_{ab} of Maxwell’s theory)."
The Weyl curvature hypothesis is that the Weyl curvature is zero at the big bang but rises gradually as the universe ages. Consequently the Weyl curvature will not be zero at black hole singularities and we may use the Weyl curvature in this picture to distinguish between cosmological singularities and other singularities. As time passes, the Weyl curvature increases and gravitational masses attract each other more strongly forming a less-homogeneous universe, with clumped masses and higher entropy encoded in the dense packing massive bodies. So that early uniform universe may be explained by there being zero Weyl curvature. Penrose talks about the Weyl curvature's growth as freeing up gravitational degrees of freedom that may then be excited. It is the excitation of these gravitaional degrees of freedom that is the real measure of entropy. It is a nice picture. But just what drives the Weyl curvature's variance is a mystery to me. It does allow us to describe gravitational entropy increase with a tensor field, and of course to associate the arrow of time with such a field. So, at least, algebraically it is appealing. It also offers an alternative to a fast period of inflation in the early universe, which some might find equally as arbitrary as a varying curvature field.
The latest idea is built upon the findings of Paul Tod in his paper "
Isotropic cosmological singularities: other matter models", where it is shown that even though the Ricci curvature blows up at the cosmological singularity the Weyl curvature remains finite. Penrose takes this finding and argues that near the big bang gravity becomes a conformal theory, so that he may rescale the metric to infinity and blow up the big bang singularity. The justification for this is that near the big bang, when temperatures are extremely high, there is little difference between the dynamics of massive and massless particles, all particles are treated as massless, and respect conformal equations of motion. Once the description of physics is conformally invariant, Penrose says that a sense of time is lost, tying in neatly with the low entropy ideas. Having blown-up the cosmological singularity, and beleiving that the Weyl curvature remains finite, has lead Penrose to ponder the smooth continuation of the Weyl curvature at the boundary. Perhaps, he suggests, in what he refers to as his "outrageous" proposal, there is a "conformal cyclic cosmology", in which one may knit the conformal geometry at the big bang to another conformal geometry prior to the big crunch, and thereby create a series of universes with a long-lived/eternal conformal geometry.
How can Penrose convince us that geometry may become conformal again at the end of the universe's lifetime? Well, he says, after most of the matter in the universe has been swallowed by black holes and has then been recycled back into the universe via massless Hawking radiation we are really only troubled by charged matter that escaped this process. Here we must presume that black holes can radiate away to pure radiation (which seems unlikely - no topology change, no unexcited microstates...) leaving a universe that may contain some unabsorbed charged matter (let's call all matter electrons) and photons. Now if we can come up with some way of doing away with the electrons, says Penrose, then we will be in business. For again without any massive particles left in the universe the scale of the metric has lost its meaning. This is the real weak point, since the mechanisms to get rid of electrons require either allowing their charge or their mass to dissipate over long time scales. But, of course, this may be possible. Once this position is arrived at one might imagine a conformal rescaling of the metric down to zero, so that a future infinite region is made finite and may be attached to the finite cosmological singularity of some other universe. Penrose argues that the appropriate conformally invariant verion of general relativity the spin-2 field picks up an inverse conformal factor when the conformal tranformation is applied to the metrc, while the Weyl curvature does not. Hence the matter density from the previous universe survives the conformal rescaling and passes over into the next universe. Penrose identifies this with the density fluctuations at the Big Bang - which is exceedingly appealing, and presumably testable.
The conformal rescaling marks the beginning of the "new" universe. In this picture there is also cosmological scale clock, whose ticks are the rescalings of the metric, so nothing to worry about on a local level. It is also imperitive that the conformal rescalings occur in the right way, i.e. to infinity at big bang singularity and to zero at late time. Effectively one must imagine that the previous universe occured at miniture scale comparatively, and the future universe will be built upon the swirling dust of ours at a gigantic scale. It doubles as a very nice picture for a science-fiction novel, as well as an exceedingly interesting proposal for the origin of the density fluctuations in the universe.
We have mentioned the assumptions, namely that black holes evaporate to pure radiation and that electron charge/mass dissipates. There are also questions about particle antiparticle pair creation, but which if we are able to argue in favour of some long term alteration of the properties of the electron, so that it eventually becomes pure radiation, this would not present a problem. Furthermore there seem to be mysterious forces driving the rescaling of the metric, for which it would seem some additional dilaton field may be necessary or some other argument presented.
You can hear Penrose talk on this in two places on the web, both of which took place at the Newton Institute. The first is from
November 2005 at the
Spitalfield's Day and the second occurred
last
week (you will have to wait until the end to hear about this cosmological model). Penrose also has written up his description of wha he refers to as "conformal cyclic cosmology" in the proceedings of the EPAC, 2006, conference, and one can read the pdf
here.
On Thursday of last week we also suffered a panel discussion on the nature of space-time, being organised by the sponsors the notorious Templeton foundation, I was a little wary. I think on the whole the event worked very well, it was simply not to

my personal taste, but I went along to enjoy the views of (Rev. Dr.)John Polkinghorne(Eclesiastical physicist), (Prof.) Shahn Majid, (Rev. Dr.) Michael Heller (of the Vatican observatory), (Sir) Roger Penrose and (Prof.) Alain Connes. As you might imagine there was a very strong representation of the religious apprecatiation of spacetime, and even Alain Connes couldn't resist talking of his interpretation of three pages of "ancient text" by which he meant the Veltman Lagrangian of the standard model. I do not think it was a night of much scientific progress. But there were some anecdotal highlights. The evening was organised so that each panelist took five minutes to mention their conception of spacetime, there was an overhead projector and it appeared that the speakers were well organised having prepared detailed slides. Throughout the first two talks Alain Connes looked a little preoccupied, occasionally staring at the desk and sometmes laying his head upon it. Suddenly after the second speaker Connes sprang to life, borrowed some OHP slides and multicoloured pens from the others, and began to prepare his own slides there and then. Since the chairman was supposedly inviting the presentations at random this seemed a wonderfully carefree approach. It made for some nice theatre. We also heard an anecdote from Roger Penrose, in response to the first question from the audience which was along the lines of 'which came first quantum mechanics or general relativity?'. Penrose replied by telling of a time he had listened to a wonderfully animated lecture by John Wheeler and at the end there came a similar question from the audience, which came first G.R. or the quantum principle? Penrose said that a small voice in the front of the audience piped up and asked 'what is the quantum principle?' The small voice belonged to Dirac. A final amusing interchange involved Shahn Majid, the chairman (Jeremy Butterfield) and a mischievous Alain Connes. Shahn Majid was summing up his disenchantment with the present understanding of spacetime with the Shakespearean line "there is something rotten in the state of Denmark" (Penrose said later he thought Majid was referring to the Copenhagen interpretation), and Alain Connes responded with another Shakespeare quotation "Throw physics to the dogs; I'll none of it." It was left to Jeremy Butterfield to point out that the actual line from Macbeth is about "physic" (referring to medecine) and not "physics". So there was some enjoyment to be had from the evening after all.